Hello, I have been trying to research how to raise the millesimal fineness of a few ounces of 835 silver I have to 925 silver. I have found previous discussions on here and the formulars don’t seem right like one says to add 40 grams of fine silver to 10 grams of 625 sliver to get 925 silver. just seem to much.
In my research I found to get 925 from fine silver (lets work with 1 toz, 31.1 grams) you divide 31.1 by .925 which gives you 33.6 so then subtracting the 31.1 gives you 2.5 grams of alloy to add to get 925.
So I thought, to get 835 silver you would do the same sum using .835 which would give you 37.2 then subtracting the 31.1 giving you 6.1 of alloy to add.
I then subtracted 2.5 off the 6.1 giving you 3.6 so in my thinking, I have to add 3.6 grams of fine silver to 31.1 grams of 835 silver to make 925 sliver. Is this right, if not, please explain why not.
Also, I am only using one decimal point here but when I go ahead, I will be using three decimal points.
Any feedback would be great,
Thanks
Dazzle.
Please correct my math where I’m wrong!
For every gram of .925 silver there is .925 g of silver and .075 g copper.
For every gram of .835 silver there is .835 g of silver and .165 g of copper.
The math is to get .165/x = .075/1 I think.
For brevity, here’s the math with wolfram alpha :
So the total weight of the material after adding pure silver would be 2.2 g. Therefore you need to add 1.2 g of pure silver to every 1 g of .835 silver to reach .925 Sterling.
Following is a link to a calculator that might help answer your question…Rob
Hi Rob, thank you for the link but it only tells you how much alloy to add to fine silver to get 925, it will come in handy when I alloy the 8 ounces of fine silver I’ve got, thanks again.
Hi Brennan,
Thanks for the input and link. I didn’t think of it that way (.075 per gram) I found that Wolfram Alpha a bit confusing, I’m not sure how you come up with 1.2 grams to every gram .835 but I find it not right. I had a look at the calculator at United Precious Metals Refining that Rob posted and found that to get .925 from 31.1 grams of fine silver, you only have to add 2.521 grams of alloy which give you 33.621 grams of .925. .835 is only .09 lower than .925, I just have to keep working on it. Thanks again
I wouldn’t think about it as 31 g right now. I think that makes it more complicated. If you think about it as 1 g then you only have to think about it as how to convert 1 g of 835 to 925.
Using that as a simplification, 835 has more than double the amount of copper as 925. Because of that you need to more than double the amount of silver to get the same quotients.
More thoughts:
We know we have to add pure silver to get the purity to .925. Because of that, we know the copper amount is not going to increase. It’s a fixed amount.
For each g of .835 silver we have .165 g of copper. That’s 2.2 times the copper amount in .925 silver. That means, if we keep the copper amount fixed at .165 g, the total amount of .925 silver will 2.2 g.
2.2 g of .925 silver has .165 g of copper in it. So all we have to do is add pure silver until we have 2.2 g of total silver.
Multiply the amount if .835 you have by 1.2 and add that much pure silver.
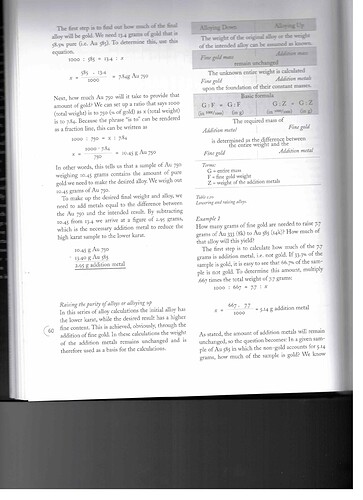
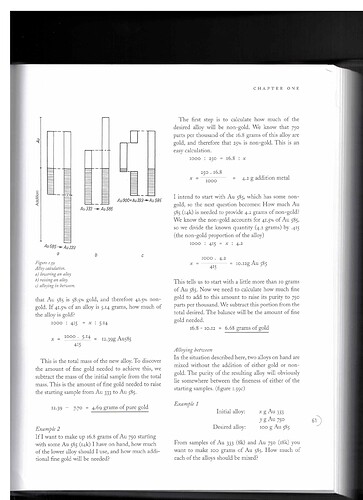
Here are two pages from Dr. Brepohl’s book that explain raising the karet of gold. Since he is dealing with parts-per-thousand using this info with silver shouldn’t be a problem.
From “The Theory and Practice of Metalsmithing” by Erhard Brepohl, posted with permission from Brynmorgen Press.
Thanks again Brennan for your input, still a little confusing but getting a handle on it (maybe I should of listen more in maths class instead of being the class clown)
A big thanks to alonzo and Brynmorgen Press for allowing you to post those pages. I’ve got my head around Example 2, still working on Example 1 but I will get there.
I liked those pages that much, I jumped on Amazon and bought a copy of the book and look forward to reading it.
Thanks again guys for all your help.
Dazzle.
I just thought of another way to wrap our heads around it because it is definitely not intuitive.
This is an abstraction so it won’t be perfectly accurate but take rice and beans.
Take 8 beans and 2 grains of rice. That signifies 80% silver and 20% copper (a rounded version of .835 silver.
Now add beans until it is 90% silver. I think what we discover is that we have to double the beans.
Anyways. It’s a fun math problem but I also found it unintuitive.
in order to get a true alloy, the atomic weights of silver and copper have to be considered. This makes it confusing. Atom percent gives you the desired alloy. Weight percent neglects the difference in the weight of pure silver and pure copper. The atomic weight of silver is 108. That of copper is 64… due to the presence of isotopes, these numbers are not 100% accurate, silver has an atomic weight of 107.868, which is close enough to 108, copper has an atomic weight of 63.546…using 64 or 63,5 makes it closer. Using atom percent, gives you the most accurate proportions of silver and copper to mix. 108 divided by 63.5 is 1.7…this ratio is the amount of copper by weight to add to 108 grams of pure silver to get any alloy based on atomic weight. Conversely the amount of pure silver that has to be added to copper to get any alloy based on atomic weight is 63.5/108… less silver than copper than by simple weight percent. If you have an alloy of 90% silver and want to bring it up to sterling, you would have to add pure silver by atomic weight. These calculations can be done for any alloy, including gold which is far heavier at 197. These ratios are used by the calculator as shown above.
The basis of atomic weight is the weight of one mole of a pure element… a mole contains 6.023 X 10 to the 23rd power of atoms… one mole of gold weighs 197 grams. a mole of silver weighs 107.87 grams and a mole of copper weighs 63.546 grams. The decimal place number are isotopes of each metal with their respective abundance. Gold has only one isotope.
Whoa there. I always assumed that percentages in alloys were by mass (weight), especially since alloys predate the mole concept by a few thousand years.
gross weight percent does not reflect the true ratio of atoms in a two component alloy. The question to ask is whether 90% silver is really 90% by atoms or actually less silver, since copper is lighter and weight percent will add more copper atoms than true 90%…True indeed that atomic weight percent is a modern chemical concept. As long as you know that 90% is not really 90%. The only alloy that you can trust is 100% is fine. not an alloy. karat gold is by mass percent… mass percent is based on atom percent. modern alloys should relfect mass percent which is molar, not simply gross weight percent.
Don’t short change yourself when buying precious metals… refiners know the difference between weight and atom percent. retailers don’t… clerks in jewerly stores except for the very high end don’t know much about alloys nor stones…
gross weight percent does not reflect the true ratio of atoms in a two component alloy. The question to ask is whether 90% silver is really 90% by atoms or actually less silver, since copper is lighter and weight percent will add more copper atoms than true 90%…
True indeed that atomic weight percent is a modern chemical concept. As long as you know that 90% is not really 90%. The only alloy that you can trust is 100% is fine. not an alloy. karat gold is by mass percent… mass percent is based on atom percent. modern alloys should relfect mass percent which is molar, not simple gross weight percent.
Don’t short change yourself when buying precious metals… refiners know the difference between weight and atom percent. retailers don’t… clerks in jewerly stores except for the very high end don’t know much about alloys nor stones…
I used to make my own solder and alloys based on weight percent. with solders, using atom percent gives better melting points… the phase diagrams between components and liquidus state are based on molar or atom%…same goes for three component alloys…
so far as gold and silver alloys are concerned, I am no longer making jewelry, I have a bunch of metal to get rid off… same with a stash of stones… getting rid of the metals is easy, but at a cost… refiners will charge 20% and return the rest in cash for the molar content of silver or gold… that’s because they have to refine it to .999% pure. One thought was to have it returned to me as investment grade bullion… for silver, there’s a $4 premium per troy ounce… the price tag becomes quite hefty when 20% refining fee is added to a $4 premium.
So far as physical gold is concerned, safety deposit boxes cost an average of $60 per month… all of the physical gold that I have other than jewerly scrap are held in a brokerage account. I can take delivery but prefer not to… I’d have to pay shipping and insurance and sell at retail… I get spot price in a brokerage account. Brokerages charge a storage fee but far below a bank safety deposit box. I bought gold bullion to take advantage of the spot price, intending to alloy it down to 18K… instead I ended up buying more as an inflation hedge… however, I recently sold half of it… gold gathers no interest nor dividends… being retired, I need something that provides income…
just to make sure that people understand: gold has underperformed the stock market over the last 40 years, substantially… in 1981, the Dow Jones average hit a mile stone of 1000… today it’s over 40K… a 40 fold increase… gold went up from $200 an ounce to $2400 today… a tenfold increase plus some… inflation over the same period of time made one dollar into 10 cents today…gold kept up with inflation but did not beat it, stocks beat inflation 4 fold…This is a digression away from the subject matter. However, it does pay to know what you are buying and selling with precious metals…especially since the price is so high…
I see. But just to make sure, as jewelers we’ll just keep doing what we’ve been doing because that’s what the customer and the market expects and understands.
of course… i’d just like to see exactly what sterling and 14K gold assays out as… customers and markets are traditional… not a problem as long as what is acceptable is stated. Gems are far far far more of a problem… synthetics are sold as “genuine”… synthetics are “genuine” in that they reproduce the chemical and crystal structure of natural but are still not natural… terminology does count.